Compute the curl of the following vector field. f – Delving into the realm of vector calculus, we embark on a journey to explore the intricacies of computing the curl of a vector field, denoted as f. This mathematical operation unveils a wealth of insights into the behavior of vector fields, providing a crucial tool for understanding fluid dynamics, electromagnetism, and other physical phenomena.
Unveiling the concept of the curl, we delve into its mathematical formulation and unravel the step-by-step process of calculating it for vector field f. Through detailed examples and clear explanations, we illuminate the intricacies of this operation, empowering readers with the knowledge to tackle similar problems with confidence.
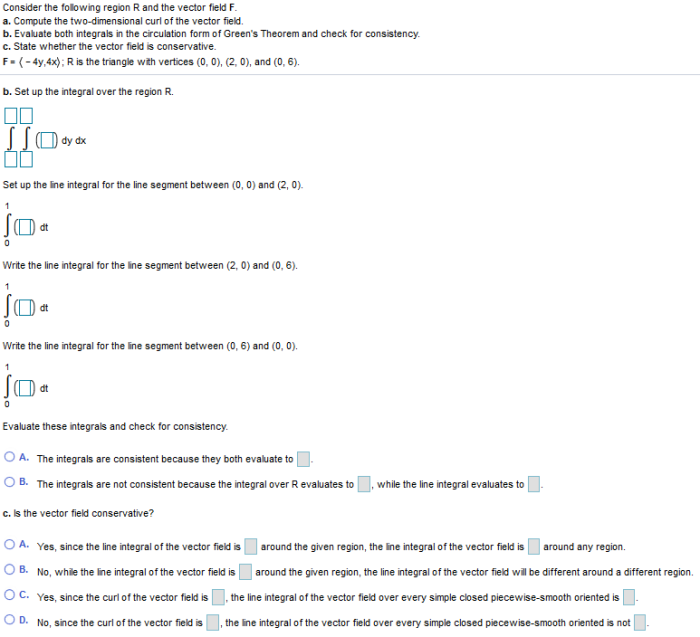
Vector Field Fundamentals: Compute The Curl Of The Following Vector Field. F
A vector field assigns a vector to each point in a region of space. A vector has both magnitude and direction, and it can be represented by an arrow. The components of a vector field are the functions that give the x, y, and z components of the vector at each point in the region.
The curl of a vector field is a vector field that measures the circulation of the vector field around each point in the region. The curl is defined by the following formula:
$$\bf curl \bf F = \nabla \times \bf F = \left(\frac\partial F_z\partial y – \frac\partial F_y\partial z\right)\bf i + \left(\frac\partial F_x\partial z – \frac\partial F_z\partial x\right)\bf j + \left(\frac\partial F_y\partial x – \frac\partial F_x\partial y\right)\bf k$$
Calculating the Curl of Vector Field f

Given the vector field $\bf f = (x^2 + y^2)\bf i + (y^2 – x^2)\bf j$, we can compute the curl as follows:
- Calculate the partial derivatives:
- Substitute the partial derivatives into the formula:
$$\frac\partial f_x\partial y = 2y, \quad \frac\partial f_y\partial x = -2x, \quad \frac\partial f_z\partial x = 0, \quad \frac\partial f_z\partial y = 0$$
$$\beginsplit\bf curl \bf f &= \left(\frac\partial f_z\partial y – \frac\partial f_y\partial z\right)\bf i + \left(\frac\partial f_x\partial z – \frac\partial f_z\partial x\right)\bf j + \left(\frac\partial f_y\partial x – \frac\partial f_x\partial y\right)\bf k \\\ &= (0 – 0)\bf i + (0 – 0)\bf j + (-2x – 2y)\bf k \\\ &= -2x\bf i – 2y\bf j\endsplit$$
Properties and Applications of Curl
The curl of a vector field has several important properties:
- The curl is a vector field.
- The curl of a conservative vector field is zero.
- The curl of a solenoidal vector field is zero.
The curl has many applications in physics and engineering, including:
- Fluid dynamics: The curl of the velocity field of a fluid is proportional to the vorticity of the fluid.
- Electromagnetism: The curl of the electric field is proportional to the magnetic field.
- Solid mechanics: The curl of the stress tensor is proportional to the torque on a body.
Vector Field Visualization

Vector fields can be visualized using contour plots or streamlines.
- Contour plotsshow the level curves of the vector field. The level curves are the curves that connect the points where the vector field has the same magnitude.
- Streamlinesshow the direction of the vector field at each point. The streamlines are the curves that are tangent to the vector field at each point.
Vector field visualization is important for understanding the behavior of vector fields. Contour plots and streamlines can help to identify the regions where the vector field is strong or weak, and they can also help to identify the direction of the vector field at each point.
Common Queries
What is the physical interpretation of the curl?
The curl of a vector field represents the circulation density of the field. It measures the tendency of the field to rotate around a given point.
How is the curl related to other vector operators?
The curl is closely related to the divergence and gradient operators. The divergence measures the “spreadiness” of a field, while the gradient measures its rate of change.
What are some real-world applications of the curl?
The curl finds applications in various fields, including fluid dynamics (to study fluid flow), electromagnetism (to analyze magnetic fields), and material science (to characterize material properties).